
Unlike line graphs, here we get a scattered set of points which are used to get ‘best fit line’ or ‘trendline’. The use of scatter plots is when there is a huge data set and the requirement is to obtain the relationship between two variables, if any exists. It is also used in finding out the future trends by studying past data. Scatter plot is one of the seven basic tools used in quality control. Each point represents one data, showing the value of both the variables for that particular data.

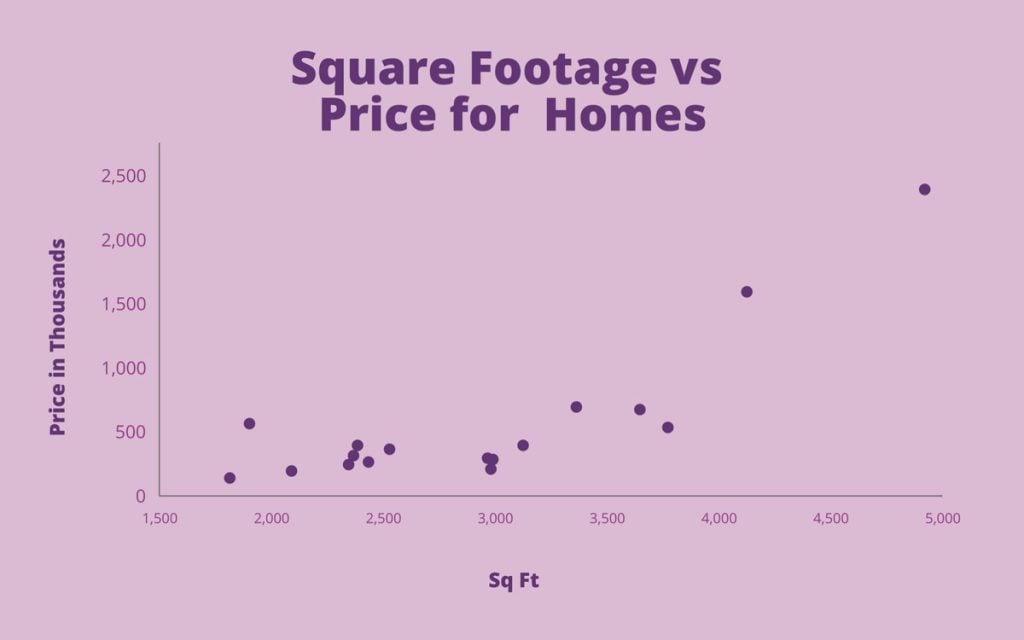
For example, a student in Year 11 has a height of 148 cm, we can estimate the arm length of this student is around 84 cm.A scatter plot, also known as scatter chart, scatter diagram, or scattergraph, is a type of mathematical chart which displays a set of data, as a collection of individual points, using two variables on the two Cartesian coordinates. This chart is very useful when one variable is easy to measure and the other is not. The chart above shows there is a positive correlation between arm length and height.Īfter determining the correlation between the variables, you can then predict the behavior of the dependent variable based on the measure of the independent variable. If we see the correlation between the student’s height and arm length exhibit a trend, we can estimate the arm length of a student with a certain value of height given and vice-versa. The scatter graph shows information about the height and the arm length of each of 8 students in Year 11.
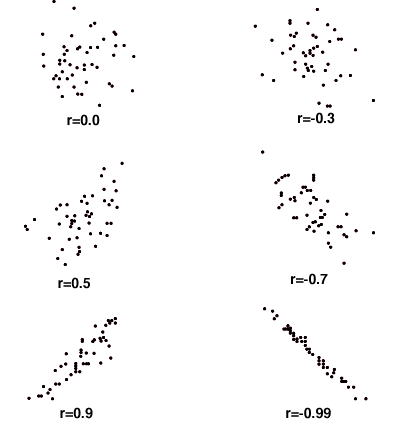
There is no demonstrated connection between the two variables.Įdit this Diagram Scatter Chart Example: Arm Length on Grade 11 The value of Y seems to be related to the value of X, but the relationship is not easily determined. The value of Y decreases slightly as the value of X increases. The value of Y increases slightly as the value of X increases. The value of Y decreases as the value of X increases. The value of Y increases as the value of X increases. The following types of scatter diagrams show in the table tell about the degree of correlation between variable X and variable Y. The degree of correlation is denoted by “r”. The more the points plotted are closer to the line, the higher is the degree of correlation. The more the points plotted are scattered over the chart, the lesser is the degree of correlation between the variables. The degree to which the variables are related to each other depends on how the points are scattered over the chart. This shows that there is a relationship between the speed of vehicles and accidents happening on the highway. Once the diagram is completed, you notice that as the speed of vehicle increases, the number of accidents also goes up. You select the two variables: motorcycle speed and number of accidents, and draw the diagram. Thus, we can use a scatter diagram to examine theories about cause-and-effect relationships and to search for root causes of an identified problem.įor example, we can analyze the pattern of motorcycle accidents on a highway. While the diagram shows relationships, it does not by itself prove that one variable causes the other.
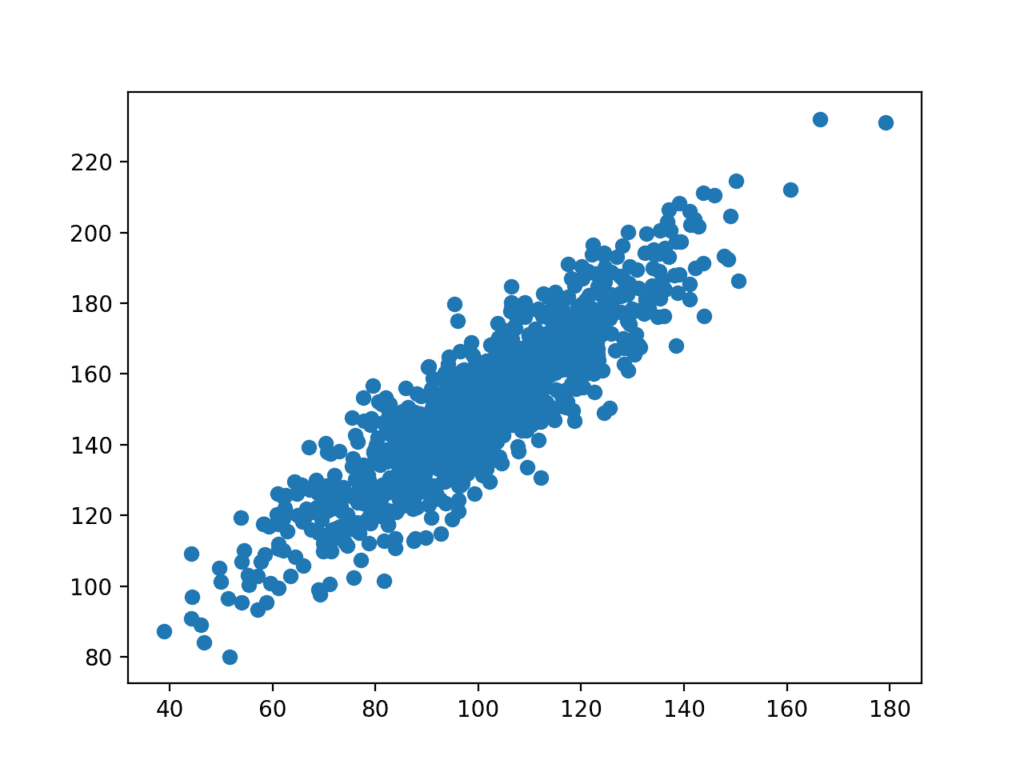
Most often a scatter diagram is used to prove or disprove cause-and-effect relationships. The pattern of their intersecting points can graphically show relationship patterns. One variable is plotted on the horizontal axis and the other is plotted on the vertical axis. View and play PowerPoint presentations online.Ī scatter diagram (Also known as scatter plot, scatter graph, and correlation chart) is a tool for analyzing relationships between two variables for determining how closely the two variables are related.


 0 kommentar(er)
0 kommentar(er)
